My Stab At Colin's Puzzle

The estimable @colinthemathmo suggests a method for estimating the radius of the earth, which he credits to a sundial expert friend named Mike:

Stand on a wall, perhaps two metres high, and wait for sunrise. When you see the sun just peak above the horizon, start the stopwatch, and jump off the wall (or drop the stopwatch to a friend). Since you are now lower down, you can no longer see the Sun, so wait a bit until you can see it again, and stop the clock.

And astonishingly, that’s enough!

When Colin says “the simplified sums are not too nasty”, you can bet you’re in for an afternoon of head-scratching. I thought it’d be interesting to do the sums. (I’m writing this in the summer, though - and while my house has a technical sea view ¹, I’m not getting up early just to test it out.)

Setting up the puzzle

So, to start with, I’m going to assume that the sun’s light shines in the plane of my circle of latitude. I’m also going to assume that I’m two metres tall, because that’s a nice number. I’m also going to assume that my circle of latitude is a lot more than 2m in radius. I’ll call that radius $r$; the time between the observations is $T$.

I’m then going to set up two right-angled triangles. The first, standing on top of the wall, has a hypotenuse of $r+4$ and a long leg of $r$. Pythagoras says that the short leg is $\sqrt{8r+16}$, which - because $r\gg 2>1$ - I’ll call $\sqrt{8r}$.

The second, from the floor, has a hypotenuse of $r+2$ and a short leg of $r$, and using similar reasoning, a short leg of $\sqrt{4r}$.

Getting into the angles

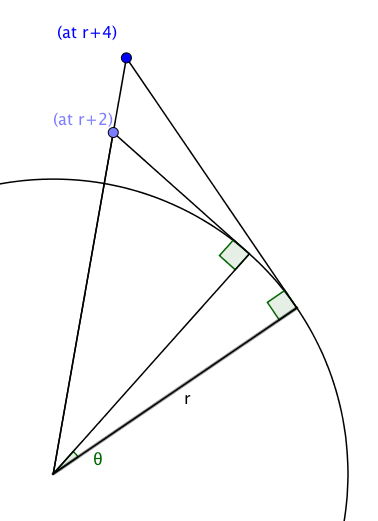
The angle turned by the earth between the two observations is the difference between the small angles in the two triangles. Supposing they’re small enough to use the small angle approximation ² $\sin (\theta )\approx \theta$ , the difference between the angles is $\theta =\frac{\sqrt{8r}}{r}-\frac{\sqrt{4r}}{r}$, or $\frac{2(\sqrt{2}-1))}{\sqrt{r}}$.

We also know, by virtue of having timed it, what fraction of a day has passed between the two observations - so $\theta =2\pi T$, measured in days.

Since $T=\frac{(\sqrt{2}-1)}{\pi \sqrt{r}}$, we can rearrange to get $r\approx {\left(\frac{(\sqrt{2}-1)}{\pi T}\right)}^2$. Switching to numbers, we get $r\approx \frac{0.0173}{T^2}$.

Adjusting for latitude

Now, since Weymouth is at 50.6º north ³, the radius of the circle of latitude is $R_E\cos \left(50.6º\right)$. That’s something like $\frac{2}{3}{R}_E$ ⁴

That gives us $R_E\approx \frac{0.0173}{0.635T^2}$, or about $\frac{0.02737}{T^2}$, where $T$ is measured in days.

Checking it’s reasonable

It’s good to go through the motions of checking that that’s a reasonable answer. After all, we know the radius of the earth: it’s about $6.4\times {10}^6$ m. Rearranging, we have $T\approx \sqrt{\frac{0.02737}{R_E}}$, which is about $\frac{0.1654}{2.5\times {10}^3}$ days, or 0.066 millidays. A centiday is about 15 minutes, which is in turn a kilosecond - so a milliday is about 100 seconds, and - assuming all is well - the time between observations ought to work out as around 7 ⁵ seconds. Does it? You can get up early and tell me. I’ve even made you a Desmos worksheet.

* Edited 2017-07-31 to fix a LaTeX error - thanks to @christianp for spotting it. * Edited 2017-07-31 to move an ambiguous footnote - thanks @colinthemathmo * Edited 2017-09-18 after @colinthemathmo spotted another error * 2020-03-27 I’m happy to provide a link to a more detailed solution by Manfred Borgens. I haven’t gone through it in detail, but Manfred has certainly put more thought into the problem than I have.

Footnotes:

1. It’s over there, between the land and the sky

2. which seems reasonable, the triangle is right-angled and pretty much isosceles

3. pretty much the only time it’s excusable to use degrees

4. $0.635R_E$, it says here.

5. Calculator work says 5.7